what to do with a negative in factoring quadratic equations
One to one maths interventions built for KS4 success
Weekly online i to i GCSE maths revision lessons now bachelor
Learn more than
This topic is relevant for:

Factorising Quadratics
Here nosotros will acquire about factorising quadratics; we volition explore what quadratic expressions are and the steps needed to factorise into double brackets.
In that location are likewise factorising quadratics worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to get next if you lot're still stuck.
First of all, let'south take a quick recap on quadratic expressions.
What is a quadratic expression?
A quadratic expression in maths is an expression including a squared term or square number i.e. a term up to
The highest power for a quadratic expression is
The general form of a quadratic expression is:
\[\color{#FE47EC}ax^{ii}\color{#00BC89}{+b}ten\color{#7C4DFF}{+c}\]
due east.g.
\[x^{2}\color{#00BC89}{-2}10\color{#7C4DFF}{+1}\]
\[\color{#FE47EC}2x^{2}\colour{#00BC89}{+iii}x\colour{#7C4DFF}{-ii}\]
We factorise quadratic expressions of this sort using double brackets. At that place are different methods we tin apply depending on whether the coefficient of
What is factorising quadratics?
Factorising, or factoring quadratic equations is the contrary of expanding brackets and is used to solve quadratic equations.
For example, in the form of
How to factorise quadratics:
- Write out the gene pairs of the last number (c).
- Notice a pair of factors that+ to give the middle number (b) and multiply to give the concluding number (c).
- Write two brackets and put the variable at the outset of each one.
- Write one cistron in the first bracket and the other factor in the 2d bracket. The gild isn't important, just the signs of the factors are.
Quadratic expressions or quadratic equations?
A quadratic equation is a quadratic expression that is equal to something. We can solve quadratic equations past using factorisation (or factoring), the quadratic formula or by completing the square.
Step past step guide: Quadratic equations

Factorising quadratics worksheets

Download two free factorising quadratics worksheets to help your students prepare for GCSEs. Includes reasoning and applied questions.
DOWNLOAD FREE

Factorising quadratics worksheets

Download two costless factorising quadratics worksheets to help your students ready for GCSEs. Includes reasoning and practical questions.
DOWNLOAD FREE
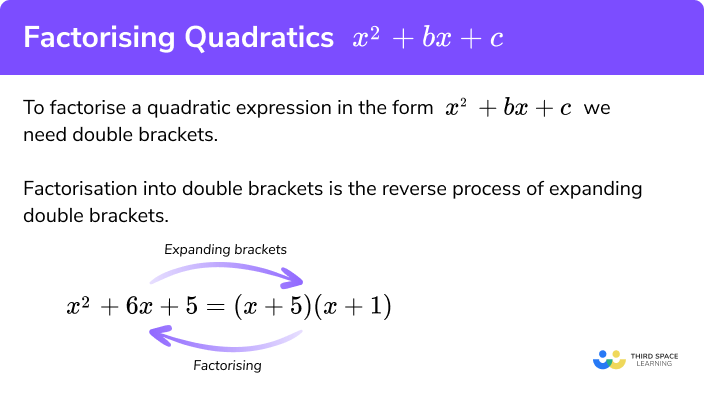
Factorising quadratics in the form ten2 + bx + c
To factorise a quadratic expression in the form
In this instance, the coefficient (number in front) of the

x2 + 6x + v = (x + 5)(ten + ane)

Explain how to factorise a quadratic expression
How to factorise quadratics: ten2 + bx + c (double brackets)
In club to factorise a quadratic algebraic expression in the form
- Write out the cistron pairs of the last number
(c) . - Notice a pair of factors that + to give the center number
(b) and ✕ to give the last number(c) . - Write two brackets and put the variable at the start of each ane.
- Write 1 cistron in the first subclass and the other cistron in the second bracket. The society isn't important, the signs of the factors are.
Factorising quadratics examples: tentwo + bx + c (double brackets)
Case 1: with +x coefficient and a + abiding
Fully factorise:
\[ten^2 \color{#00BC89}{+ 6x}\colour{#7C4DFF} {+ v}\]
- Write out the factor pairs of the terminal number (5) in order.
\[x^2 \color{#00BC89}{+ 6x}\color{#7C4DFF} {+ v}\]
Factors of 5:
one, 5
2We need a pair of factors that + to requite the heart number (vi) and ✕ to give the terminal number (5).
\[x^2 \color{#00BC89}{+ 6x}\color{#7C4DFF} {+ 5}\]
Factors of 5:
ane, 5
(It'southward a good idea to exercise a quick check that we have the correct numbers)
Retrieve: to multiply two values together to give a positive answer, the signs must be the aforementioned
3Write ii brackets and put the variable at the kickoff of each one (x in this case).
\[(x\qquad)(x\qquad)\]
ivWrite one factor in the first bracket and the other gene in the second bracket. The social club isn't important, the signs of the factors are.
\[(x+\color{#FF9100}one)(x+\colour{#FF9100}five)\]
We have at present fully factorised the quadratic expression.
Nosotros can check the respond by multiplying out the brackets!
\[(x+1)(x+5)=ten^{ii}+6x+5\]
Example 2: with -10 coefficient and a – constant
Fully factorise:
\[10^2 – 2x – 24\]
Write out the gene pairs of the last number (24) in guild
Factors of 24:
1, 24
2, 12
3, 8
4, 6
We demand a pair of factors that + to give the heart number (-two) and ✕ to give the last number (-24).
Factors of 24:
i, 24
ii, 12
3, eight
4, 6
-6 + iv = -2 ✔
-6 ✕ 4 = -24 ✔
(It's a practiced thought to do a quick check that we have the right numbers)
Remember: to multiply two values together to give a negative answer, the signs must be the different.
Write two brackets and put the variable at the start of each one (x in this example).
Write one cistron in the first subclass and the other factor in the 2d subclass. The order isn't important, the signs of the factors are.
Nosotros have at present fully factorised the quadratic expression.
We can bank check the answer by multiplying out the brackets!
Example iii: with +ten coefficient and a – constant
Fully factorise:
Write out the cistron pairs of final number (20) in order.
\[10^{2}+x-20\]
Factors of 20:
1, 20
two, 10
iv, five
We demand a pair of factors that + to give the middle number (i) and ✕ to give the last number (-20).
Factors of 20:
1, twenty
two, 10
4, 5
-four + v = ane ✔
-iv ✕ 5 = -20 ✔
(It'due south a proficient idea to do a quick check that nosotros have the correct numbers)
Remember: to ✕ ii values together to give a negative reply, the signs must exist the different
Write 2 brackets and put the variable at the start of each ane (x in this instance)
Write one factor in the first bracket and the other factor in the second bracket. The order isn't important, the signs of the factors are.
We can check the reply by multiplying out the brackets!
Example 4: with -10 coefficient and a + constant
Fully factorise:
\[10^2 – 8x + 15\]
Write out the cistron pairs of the terminal number (15) in club.
Factors of 15:
one, fifteen
3, 5
We need a pair of factors that + to requite the middle number (-8) and ✕ to give the last number (fifteen).
Factors of 15:
1, fifteen
3, v
-three + -5 = -8 ✔
-three ✕ -5 = 15 ✔
It'due south a expert idea to practice a quick check that we have the correct numbers.
Remember: to ✕ two values together to give a positive answer, the signs must exist the aforementioned
Write two brackets and put the variable at the commencement of each one (x in this example)
Write one factor in the showtime bracket and the other factor in the second subclass.
\[(x – iii)(ten – 5)\]
We have now fully factorised the quadratic expression.
We tin can bank check the answer by multiplying out the brackets!
Practice factorising quadratics questions: x2 + bx + c (double brackets)




Nosotros need numbers that take a product of 6 and a sum of 5 . By considering the factors of vi , we conclude that x^{2}+5x+6=(x+3)(x+2) .




We need numbers that have a product of 21 and a sum of ten . By considering the factors of 21 , we conclude that ten^{2}+10x+21=(x+3)(x+7) .




We need numbers that have a product of -12 and a sum of -1 . Past because the factors of -12 , we conclude that 10^{2}-ten-12=(x-4)(x+3) .




Nosotros need numbers that have a product of -18 and a sum of 3 . By because the factors of -eighteen , we conclude that x^{two}+3x-18=(x+6)(ten-3) .




We demand numbers that have a product of 8 and a sum of -6 . By because the negative factors of 8 , we conclude that x^{ii}-6x+viii=(x-2)(x-4) .




Nosotros need numbers that have a production of 24 and a sum of -10 . By considering the negative factors of 24 , nosotros conclude that 10^{ii}-10x+24=(10-4)(10-6) .
Factorising quadratics GCSE questions: ten2 + bx + c (double brackets)
ane. Factorise: 102 + 3x – 10
Show respond
(2 marks)
2. Factorise: y2 – 10y + 16
Show respond
(2 marks)
iii. Factorise: tentwo – 12x + 27
Show answer
(2 marks)
Factorising quadratics in the form ax2 + bx + c
To factorise a quadratic expression in the form
In this case the coefficient (number in front) of the

2x2 + 5x + iii = (2x + 3)(x + 1)

How to factorise quadratics: ax2 + bx + c (double brackets)
In order to factorise a quadratic algebraic expression in the grade
- Multiply the cease numbers together (
a andc ) and so write out the gene pairs of this new number in order. - Nosotros need a pair of factors that + to requite the center number (
b ) and ✕ to give this new number. - Rewrite the original expression, this fourth dimension splitting the middle term into the two factors we found in stride ii. The order of these factors doesn't matter, the signs do.
- Separate the equation downwards the middle and fully factorise each half. The expressions in the brackets must be the same!
- Factorise the whole expression by bringing the contents of the bracket to the front end and writing the two other terms in the other bracket.
Factorising quadratics examples: axii + bx + c (double brackets)
Example 1: with +x coefficient and a + constant
Fully factorise:
\[2x^2 + 5x + 3\]
- Multiply the end numbers together (ii and three) and so write out the factor pairs of this new number in society.
Factors of vi:
1, half-dozen
2, 3
2We need a pair of factors that + to give the middle number (
Factors of half dozen:
1, six
2, 3
+ 5
✕ 6
2 + 3 = five ✔
2 10 3 = 6 ✔
Remember: to ten ii values together to give a positive answer, the signs must be the same.
3Go back to the original equation and rewrite it this time splitting the middle term into the two factors we found in stride 2 – the club of these factors doesn't matter, the signs do.
2x2 + 5x + 3
2xtwo + 2x + 3x + iii
ivSplit the equation downwards the centre into two halves and fully factorise each half – the expressions in the brackets must be the same!
2x2 + 5x + 3
2x2 + 2x + 3x + iii
2x(x + 1) + iii(x + 1)
5Now factorise the whole expression by bringing whatever is in the bracket to the front and writing the two other terms in the other bracket.
(ten + one)(2x + 3)
The club of the brackets doesn't thing
We take now fully factorised the quadratic expression.
We can bank check the answer by multiplying out the brackets!
Example 2: with +x coefficient and a – constant
Fully factorise:
\[2x^2 + 3x – 2\]
Multiply the the end numbers together (2 and -2) then write out the factor pairs of this new number in order.
Factors of 4:
1, 4
ii, 2
We need a pair of factors that + to give the middle number (iii) and ✕ to give this new number (-4)
Factors of four:
1, 4
2, 2
⊕ 3
✕ -4
-1 + 4 = 3 ✔
-i ✕ four = -four ✔
Recollect: to x two values together to give a negative reply, the signs must be different
Go back to the original equation and rewrite it this fourth dimension splitting the middle term into the two factors we found in step 2 – the order of these factors doesn't matter, the signs do.
2xtwo + 3x - 2
2x2 - 10 + 4x - 2
Split the equation down the centre into 2 halves and fully factorise each half – the expressions in the brackets must be the same!
2x2 + 3x - 2 2xii - x + 4x - 2 x(2x + 1) + two(2x - 1)
Now factorise the whole expression by bringing whatever is in the bracket to the front and writing the two other terms in the other subclass.
We have now fully factorised the quadratic expression.
We can check the reply past multiplying out the brackets!
Instance 3: with -x coefficient and a – constant
Fully factorise:
\[3x^2 – 2x – 8\]
Multiply the the end numbers together (three and -8) and so write out the factor pairs of this new number in order.
Factors of 24:
1, 24
ii, 12
three, 8
4, 6
We need a pair of factors that + to give the middle number (-2) and to ✕ give this new number (-24)
Factors of 24:
1, 24
2, 12
iii, viii
iv, 6
⊕ -2
✕ -24
-6 + 4 = -ii ✔
-6 ✕ four = -24 ✔
Call back: to ✕ two values together to give a negative answer, the signs must be dissimilar
Go dorsum to the original equation and rewrite information technology this time splitting the centre term into the 2 factors we found in step two – the order of these factors doesn't matter, the signs do.
3x2 - 2x - 8
3xii - 6x + 4x - 8
Split the equation down the middle into two halves and fully factorise each one-half – the expressions in the brackets must be the same!
3x2 - 2x - 8
3xtwo - 6x + 4x - 8
3x(x - two) + 4(x - two)
At present factorise the whole expression by bringing whatsoever is in the subclass to the front and writing the 2 other terms in the other subclass.
We take at present fully factorised the quadratic expression.
We can cheque the reply by multiplying out the brackets!
Example 4: with -x coefficient and a + constant
Fully factorise:
\[ 6x^2 – 7x + two \]
Multiply the the stop numbers together (6 and 2) and so write out the factor pairs of this new number in order.
Factors of 12:
ane, 12
2, 6
3, iv
We need a pair of factors that + to give the heart number (-vii) and ✕ to requite this new number (12)
Factors of 12:
1, 12
two, 6
3, iv
+ -vii
✕ -24
-three + -4 = -7 ✔
-three ✕ -4 = 12 ✔
Recollect: to ✕ two values together to requite a positive respond, the signs must be the same
Become back to the original equation and rewrite it this time splitting the middle term into the two factors we found in step two – the lodge of these factors doesn't matter, the signs do.
6xii - 7x + 2
6xtwo - 3x - 4x + two
Split the equation down the centre into two halves and fully factorise each half – the expressions in the brackets must exist the same!
6xtwo - 7x + two
6x2 - 3x - 4x + 2
3x(2x - ane) - 2(2x - 1)
Now factorise the whole expression by bringing any is in the bracket to the front and writing the two other terms in the other bracket.
Nosotros accept now fully factorised the quadratic expression.
Nosotros tin check the answer by multiplying out the brackets!
Practice factorising quadratics questions: ax2 + bx + c (double brackets)




Using the method from the above lesson, nosotros can rewrite 2x^{2}+5x+2 equally 2x^{2}+4x+x+2 which tin be factorised as 2x(10+2)+1(x+2) or more concisely, (2x+1)(x+2) .




Using the method from the above lesson, we tin can rewrite 2x^{2}+x-6 as 2x^{2}-3x+4x-6 which tin can exist factorised as 10(2x-3)+two(2x-3) or more concisely, (2x-3)(x+2) .




For the given expression, 2x^{2}-14x+20 first cistron out the HCF of 2 , giving two[x^{2}-7x+10] then apply the method from the lesson to factorise this simpler expression, hence two[x^{2}-5x-2x+x] or two[10(10-5)-2(x-5)] so that the fully factorised expression is 2(x-ii)(x-5) .




Using the method from the above lesson, we can rewrite 3x^{ii}-7x-six every bit 3x^{2}-9x+2x-6 which tin be factorised as 3x(x-3)+2(10-three) or more concisely, (3x+2)(x-3) .




Using the method from the above lesson, we can rewrite 3x^{2}-7x+2 as 3x^{2}-6x-x+two which can exist factorised as 3x(x-2)-(x-2) or more concisely, (3x-1)(x-2) .




For the given expression, 4x^{ii}-18x+viii beginning cistron out the HCF of 2 , giving 2(2x^{2}-9x+4) so apply the method from the lesson to factorise this simpler expression, hence 2[2x^{2}-8x-x+4] or 2[2x(10-4)-(x-4)] so that the fully factorised expression is ii(2x-1)(x-4) .
Factorising quadratics GCSE questions: axii + bx + c (double brackets)
1. Factorise: 2x2 + 9x + iv
Prove respond
(2 marks)
2. Factorise: 2y2 – y – 3
Evidence answer
(2 marks)
three. Factorise: 2x2 – ten – 10
Show answer
(2 marks)
Mutual misconceptions
- The order of the brackets
When nosotros multiply two values the order doesn't matter. This is true for the brackets when factorising quadratics
e.one thousand.
2 ✕ 3 = 3 ✕ two Information technology is exactly the same here.
(x – half-dozen)(x + 4) means(x – 6)(x + 4) So,
(x – 6)(10 + 4)=(10 + four)(x – vi)
- The signs of the factors
It is mutual for students to become confused regarding the signs of the factors in the brackets, particularly with negative factors.eastward.g. if the factors are
-6 and4 , the numbers is the brackets must be:
(x – 6)(x + 4)
- Multiplying ii numbers to requite a +
For two numbers to multiply to give a + their signs must be the aforementioned, that is a negative x a negative or a positive x a positive.
+ ✕ + = +
east.thousand 2 ✕ 3 = six
four ✕ 5=20
– ✕ – = +
e.g -2 ✕ -3 = 6
-iv ✕ -5= 20
- Multiplying two numbers to give a –
For two numbers to multiply to requite a – their signs must exist dissimilar, that is a negative x a positive or a positive ten a negative.
+ ✕ – = –
due east.k 2 ✕ -3= -vi
4 ✕ -v= -20
– ✕ + = –
east.one thousand -two ✕ iii= -six
-4 ✕ 5= -xx
- The signs are of import for the stop numbers too
eastward.thou. 3x^{2} - 2x - 8
three x -eight = -24 NOT 24
- Meet likewise: Negative numbers
Learning checklist
You have now learnt how to:
-
Dispense algebraic expressions by taking out common factors to factorise into a single bracket - Factorise quadratic expressions of the form x^{2} + bx + c
-
Factorise quadratic expressions of the class of the deviation of two squares - Factorising quadratic expressions of the class ax^{2} + bx + c (H)
Nevertheless stuck?
Prepare your KS4 students for maths GCSEs success with Third Infinite Learning. Weekly online one to one GCSE maths revision lessons delivered by skilful maths tutors.

Find out more about our GCSE maths revision program.
We use essential and non-essential cookies to improve the feel on our website. Please read our Cookies Policy for information on how we utilise cookies and how to manage or change your cookie settings.Accept
Source: https://thirdspacelearning.com/gcse-maths/algebra/factorising-quadratics/
0 Response to "what to do with a negative in factoring quadratic equations"
Postar um comentário